When solving geometric problems, it is useful to follow such an algorithm. While reading the conditions of the problem, it is necessary

• Make a drawing. The drawing should correspond as much as possible to the conditions of the problem, so its main task is to help find the solution
• Put all the data from the problem statement on the drawing
• Write down all the geometric concepts that appear in the problem
• Remember all the theorems that relate to these concepts
• Draw on the drawing all the relationships between the elements of a geometric figure that follow from these theorems

For example, if the problem contains the words bisector of an angle of a triangle, you need to remember the definition and properties of a bisector and indicate equal or proportional segments and angles in the drawing.

In this article you will find the basic properties of a triangle that you need to know to successfully solve problems.

TRIANGLE.

Area of ​​a triangle.

1. ,

here - an arbitrary side of the triangle, - the height lowered to this side.

2. ,

here and are arbitrary sides of the triangle, and is the angle between these sides:

3. Heron's formula:

Here are the lengths of the sides of the triangle, is the semi-perimeter of the triangle,

4. ,

here is the semi-perimeter of the triangle, and is the radius of the inscribed circle.

Let be the lengths of the tangent segments.

Then Heron's formula can be written as follows:

5.

6. ,

here - the lengths of the sides of the triangle, - the radius of the circumscribed circle.

If a point is taken on the side of a triangle that divides this side in the ratio m: n, then the segment connecting this point with the vertex of the opposite angle divides the triangle into two triangles, the areas of which are in the ratio m: n:

The ratio of the areas of similar triangles is equal to the square of the similarity coefficient.

Median of a triangle

This is a segment connecting the vertex of a triangle to the middle of the opposite side.

Medians of a triangle intersect at one point and are divided by the intersection point in a ratio of 2:1, counting from the vertex.

The intersection point of the medians of a regular triangle divides the median into two segments, the smaller of which is equal to the radius of the inscribed circle, and the larger of which is equal to the radius of the circumscribed circle.

The radius of the circumscribed circle is twice the radius of the inscribed circle: R=2r

Median length arbitrary triangle

,

here - the median drawn to the side - the lengths of the sides of the triangle.

Bisector of a triangle

This is the bisector segment of any angle of a triangle connecting the vertex of this angle with the opposite side.

Bisector of a triangle divides a side into segments proportional to the adjacent sides:

Bisectors of a triangle intersect at one point, which is the center of the inscribed circle.

All points of the angle bisector are equidistant from the sides of the angle.

Triangle height

This is a perpendicular segment dropped from the vertex of the triangle to the opposite side, or its continuation. In an obtuse triangle, the altitude drawn from the vertex of the acute angle lies outside the triangle.

The altitudes of a triangle intersect at one point, which is called orthocenter of the triangle.

To find the height of a triangle drawn to the side, you need to find its area in any available way, and then use the formula:

Center of the circumcircle of a triangle, lies at the intersection point of the perpendicular bisectors drawn to the sides of the triangle.

Circumference radius of a triangle can be found using the following formulas:

Here are the lengths of the sides of the triangle, and is the area of ​​the triangle.

,

where is the length of the side of the triangle and is the opposite angle. (This formula follows from the sine theorem.)

Triangle inequality

Each side of the triangle is less than the sum and greater than the difference of the other two.

The sum of the lengths of any two sides is always greater than the length of the third side:

Opposite the larger side lies the larger angle; Opposite the larger angle lies the larger side:

If , then vice versa.

Theorem of sines:

The sides of a triangle are proportional to the sines of the opposite angles:

Cosine theorem:

The square of a side of a triangle is equal to the sum of the squares of the other two sides without twice the product of these sides by the cosine of the angle between them:

Right triangle

- This is a triangle, one of the angles of which is 90°.

The sum of the acute angles of a right triangle is 90°.

The hypotenuse is the side that lies opposite the 90° angle. The hypotenuse is the longest side.

Pythagorean theorem:

the square of the hypotenuse is equal to the sum of the squares of the legs:

The radius of a circle inscribed in a right triangle is equal to

,

here is the radius of the inscribed circle, - the legs, - the hypotenuse:

Center of the circumcircle of a right triangle lies in the middle of the hypotenuse:

Median of a right triangle drawn to the hypotenuse, is equal to half the hypotenuse.

Definition of sine, cosine, tangent and cotangent of a right triangle look

The ratio of elements in a right triangle:

The square of the altitude of a right triangle drawn from the vertex of a right angle is equal to the product of the projections of the legs onto the hypotenuse:

The square of the leg is equal to the product of the hypotenuse and the projection of the leg onto the hypotenuse:

Leg lying opposite the corner equal to half the hypotenuse:

Isosceles triangle.

The bisector of an isosceles triangle drawn to the base is the median and altitude.

In an isosceles triangle, the base angles are equal.

Apex angle.

And - sides,

And - angles at the base.

Height, bisector and median.

Attention! The height, bisector and median drawn to the side do not coincide.

Regular triangle

(or equilateral triangle ) is a triangle, all sides and angles of which are equal to each other.

Area of ​​a regular triangle equal to

where is the length of the side of the triangle.

Center of a circle inscribed in a regular triangle, coincides with the center of the circle circumscribed about a regular triangle and lies at the point of intersection of the medians.

Intersection point of the medians of a regular triangle divides the median into two segments, the smaller of which is equal to the radius of the inscribed circle, and the larger of which is equal to the radius of the circumscribed circle.

If one of the angles of an isosceles triangle is 60°, then the triangle is regular.

Middle line of the triangle

This is a segment connecting the midpoints of two sides.

In the figure DE is the middle line of triangle ABC.

The middle line of the triangle is parallel to the third side and equal to its half: DE||AC, AC=2DE

External angle of a triangle

This is the angle adjacent to any angle of the triangle.

An exterior angle of a triangle is equal to the sum of two angles not adjacent to it.

External angle trigonometric functions:

Signs of equality of triangles:

1 . If two sides and the angle between them of one triangle are respectively equal to two sides and the angle between them of another triangle, then such triangles are congruent.

2 . If a side and two adjacent angles of one triangle are respectively equal to a side and two adjacent angles of another triangle, then such triangles are congruent.

3 If three sides of one triangle are respectively equal to three sides of another triangle, then such triangles are congruent.

Important: since in a right triangle two angles are obviously equal, then for equality of two right triangles equality of only two elements is required: two sides, or a side and an acute angle.

Signs of similarity of triangles:

1 . If two sides of one triangle are proportional to two sides of another triangle, and the angles between these sides are equal, then these triangles are similar.

2 . If three sides of one triangle are proportional to three sides of another triangle, then the triangles are similar.

3 . If two angles of one triangle are equal to two angles of another triangle, then the triangles are similar.

Important: In similar triangles, similar sides lie opposite equal angles.

Menelaus' theorem

Let a line intersect a triangle, and is the point of its intersection with side , is the point of its intersection with side , and is the point of its intersection with the continuation of side . Then

A certain triangle in which all sides are not of the same length is usually called versatile.

A triangle with two equal sides is denoted as isosceles. Identical sides are usually called lateral, third party - basis. The following definition will be equally true triangle bases is the side of an isosceles triangle that is not equal to the other two sides.

IN isosceles triangle the angles at the base are equal. Height, median, bisector of an isosceles triangle, drawn to its base, are aligned.

Triangle, with all equal sides, is denoted as equilateral or correct. In an equilateral triangle, all angles are 60°, and the centers of the inscribed and circumscribed circles are aligned.

Types of triangles depending on angle parameters.

A triangle in which only angles less than 90 0 (acute) is called acute-angled.

A triangle containing an angle of 90 0 is called rectangular. The sides of a triangle forming a right angle are usually designated legs, and the side opposite the right angle is hypotenuse.

Today we are going to the country of Geometry, where we will get acquainted with different types of triangles.

Consider the geometric shapes and find the “extra” one among them (Fig. 1).

Rice. 1. Illustration for example

We see that figures No. 1, 2, 3, 5 are quadrilaterals. Each of them has its own name (Fig. 2).

Rice. 2. Quadrilaterals

This means that the “extra” figure is a triangle (Fig. 3).

Rice. 3. Illustration for example

A triangle is a figure that consists of three points that do not lie on the same line and three segments connecting these points in pairs.

The points are called vertices of the triangle, segments - his parties. The sides of the triangle form There are three angles at the vertices of a triangle.

The main features of a triangle are three sides and three corners. According to the size of the angle, triangles are acute, rectangular and obtuse.

A triangle is called acute-angled if all three of its angles are acute, that is, less than 90° (Fig. 4).

Rice. 4. Acute triangle

A triangle is called rectangular if one of its angles is 90° (Fig. 5).

Rice. 5. Right Triangle

A triangle is called obtuse if one of its angles is obtuse, that is, more than 90° (Fig. 6).

Rice. 6. Obtuse triangle

Based on the number of equal sides, triangles are equilateral, isosceles, scalene.

An isosceles triangle is one in which two sides are equal (Fig. 7).

Rice. 7. Isosceles triangle

These sides are called lateral, third party - basis. In an isosceles triangle, the base angles are equal.

There are isosceles triangles acute and obtuse(Fig. 8) .

Rice. 8. Acute and obtuse isosceles triangles

An equilateral triangle is one in which all three sides are equal (Fig. 9).

Rice. 9. Equilateral triangle

In an equilateral triangle all angles are equal. Equilateral triangles Always acute-angled.

A scalene triangle is one in which all three sides have different lengths (Fig. 10).

Rice. 10. Scalene triangle

Complete the task. Distribute these triangles into three groups (Fig. 11).

Rice. 11. Illustration for the task

First, let's distribute according to the size of the angles.

Acute triangles: No. 1, No. 3.

Right triangles: No. 2, No. 6.

Obtuse triangles: No. 4, No. 5.

We will distribute the same triangles into groups according to the number of equal sides.

Scalene triangles: No. 4, No. 6.

Isosceles triangles: No. 2, No. 3, No. 5.

Equilateral triangle: No. 1.

Look at the pictures.

Think about what piece of wire each triangle was made from (Fig. 12).

Rice. 12. Illustration for the task

You can think like this.

The first piece of wire is divided into three equal parts, so you can make an equilateral triangle from it. He is shown third in the picture.

The second piece of wire is divided into three different parts, so it can be used to make a scalene triangle. It is shown first in the picture.

The third piece of wire is divided into three parts, where two parts have the same length, which means that an isosceles triangle can be made from it. In the picture he is shown second.

Today in class we learned about different types of triangles.

References

1. M.I. Moreau, M.A. Bantova and others. Mathematics: Textbook. 3rd grade: in 2 parts, part 1. - M.: “Enlightenment”, 2012.
2. M.I. Moreau, M.A. Bantova and others. Mathematics: Textbook. 3rd grade: in 2 parts, part 2. - M.: “Enlightenment”, 2012.
3. M.I. Moro. Math lessons: Methodical recommendations for the teacher. 3rd grade. - M.: Education, 2012.
4. Regulatory document. Monitoring and evaluation of learning outcomes. - M.: “Enlightenment”, 2011.
5. "School of Russia": Programs for primary school. - M.: “Enlightenment”, 2011.
6. S.I. Volkova. Mathematics: Test work. 3rd grade. - M.: Education, 2012.
7. V.N. Rudnitskaya. Tests. - M.: “Exam”, 2012.

1. Nsportal.ru ().
2. Prosv.ru ().
3. Do.gendocs.ru ().

Homework

1. Complete the phrases.

a) A triangle is a figure that consists of ... that do not lie on the same line, and ... that connect these points in pairs.

b) The points are called … , segments - his … . The sides of the triangle form at the vertices of the triangle ….

c) According to the size of the angle, triangles are ... , ... , ... .

d) Based on the number of equal sides, triangles are ... , ... , ... .

2. Draw

a) right triangle;

b) acute triangle;

c) obtuse triangle;

d) equilateral triangle;

e) scalene triangle;

e) isosceles triangle.

3. Create an assignment on the topic of the lesson for your friends.

With the smallest number of corners and sides. A triangle is formed by a closed broken line, consisting of three links, and that part of the plane that is inside the broken line.

In the text, triangles are designated by the symbol Δ and three capital Latin letters at the vertices - Δ ABC:

In a triangle ABC points A, B And C- This vertices of the triangle, segments AB, B.C. And C.A. - sides of the triangle. The angles formed by the sides of a triangle are called angles of the triangle.

The bottom side of the triangle is usually called basis. In a triangle ABC side A.C.- base.

Types of triangles

Triangles differ from each other, firstly, by the nature of the angles, and secondly, by the nature of the sides.

Based on the nature of the angles, a triangle is called:

• Acute-angled, if all its angles are acute.
• Rectangular if one angle is right. In a right triangle, the sides forming a right angle are called legs, and the side lying opposite the right angle is hypotenuse.
• Obtuse, if one of its angles is obtuse.

Based on the nature of the sides, a triangle is called:

• Versatile, if all its sides have different lengths.
• Isosceles, if its two sides are equal to each other. Equal sides are called sides, and the third party - basis. In isosceles triangles, the base angles are equal.
• Equilateral, if all three of its sides are equal to each other. In equilateral triangles, all three angles are equal.

Equal sides of the side in the drawings are marked with the same number of lines.

Triangle . Acute, obtuse and right triangle.

Legs and hypotenuse. Isosceles and equilateral triangle.

Sum of angles of a triangle.

External angle of a triangle. Signs of equality of triangles.

Remarkable lines and points in a triangle: heights, medians,

bisectors, median e perpendiculars, orthocenter,

center of gravity, center of a circumscribed circle, center of an inscribed circle.

Pythagorean theorem. Aspect ratio in an arbitrary triangle.

Triangle is a polygon with three sides (or three angles). The sides of a triangle are often indicated by small letters that correspond to the capital letters representing the opposite vertices.

If all three angles are acute (Fig. 20), then this is acute triangle . If one of the angles is right(C, Fig.21), then this right triangle; sidesa , bforming a right angle are called legs; sidec, opposite right angle, called hypotenuse. If one of obtuse angles (B, Fig. 22), then this obtuse triangle.


Triangle ABC (Fig. 23) - isosceles, If two its sides are equal (a= c); these equal sides are called lateral, the third party is called basis triangle. Triangle ABC (Fig. 24) – equilateral, If All its sides are equal (a = b = c). In general case ( a ≠ b ≠ c) we have scalene triangle .

Basic properties of triangles. In any triangle:

1. Opposite the larger side lies the larger angle, and vice versa.

2. Equal angles lie opposite equal sides, and vice versa.

In particular, all angles in equilateral triangle are equal.

3. The sum of the angles of a triangle is 180 º .

From the last two properties it follows that every angle in an equilateral

triangle is 60 º.

4. Continuing one of the sides of the triangle (AC, Fig. 25), we get external

angle BCD . The external angle of a triangle is equal to the sum of the internal angles,

not adjacent to it : BCD = A + B.

5. Any side of a triangle is less than the sum of the other two sides and greater

their differences (a < b + c, a > b – c;b < a + c, b > a – c;c < a + b,c > a – b).

Signs of equality of triangles.

Triangles are congruent if they are respectively equal:

a ) two sides and the angle between them;

b ) two corners and the side adjacent to them;

c) three sides.

Signs of equality of right triangles.

Two rectangular triangles are equal if one of the following conditions is met:

1) their legs are equal;

2) the leg and hypotenuse of one triangle are equal to the leg and hypotenuse of the other;

3) the hypotenuse and acute angle of one triangle are equal to the hypotenuse and acute angle of the other;

4) the leg and the adjacent acute angle of one triangle are equal to the leg and the adjacent acute angle of the other;

5) the leg and the opposite acute angle of one triangle are equal to the leg and the opposite acute angle of the other.

Wonderful lines and points in the triangle.

Height triangle isperpendicular,lowered from any vertex to the opposite side ( or its continuation). This side is calledbase of the triangle . The three altitudes of a triangle always intersectat one point, called orthocenter triangle. Orthocenter of an acute triangle (point O , Fig. 26) is located inside the triangle, andorthocenter of an obtuse triangle (point O , fig.27) – outside; The orthocenter of a right triangle coincides with the vertex of the right angle.

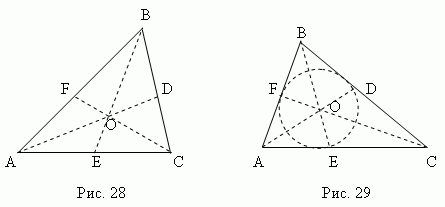
Median - This segment , connecting any vertex of a triangle to the middle of the opposite side. Three medians of a triangle (AD, BE, CF, fig.28) intersect at one point O , always lying inside the triangle and being his center of gravity. This point divides each median in a ratio of 2:1, counting from the vertex.

Bisector - This bisector segment angle from vertex to point intersections with the opposite side. Three bisectors of a triangle (AD, BE, CF, fig. 29) intersect at one point Oh, always lying inside the triangle And being center of the inscribed circle(see section “Inscribedand circumscribed polygons").

The bisector divides the opposite side into parts proportional to the adjacent sides ; for example, in Fig. 29 AE: CE = AB: BC.

Median perpendicular is a perpendicular drawn from the middle segment points (sides). Three perpendicular bisectors of triangle ABC(KO, MO, NO, Fig. 30 ) intersect at one point O, which is center circumscribed circle (points K, M, N – the midpoints of the sides of the triangle ABC).

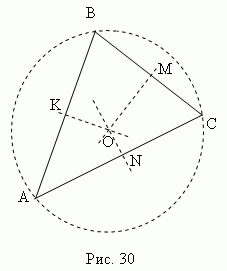
In an acute triangle, this point lies inside the triangle; in obtuse - outside; in a rectangular - in the middle of the hypotenuse. Orthocenter, center of gravity, circumcenter and inscribed circle coincide only in an equilateral triangle.

Pythagorean theorem. In a right triangle, the square of lengthThe hypotenuse is equal to the sum of the squares of the lengths of the legs.

The proof of the Pythagorean theorem follows clearly from Fig. 31. Consider a right triangle ABC with legs a , b and hypotenuse c.

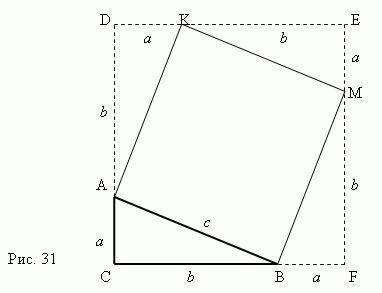
Let's build a square AKMB using the hypotenuse AB as a side. Thencontinue the sides of the right triangle ABC so as to get a square CDEF , whose side is equala + b .Now it is clear that the area of ​​the square CDEF is equal to ( a+b) 2 . On the other hand, this area equals the sum areas four right triangles and the square AKMB, that is

c 2 + 4 (ab / 2) = c 2 + 2 ab,

from here,

c 2 + 2 ab= (a+b) 2 ,

and finally we have:

c 2 =a 2 +b 2 .

Aspect ratio in an arbitrary triangle.

In the general case (for an arbitrary triangle) we have:

c 2 =a 2 +b 2 – 2ab· cos C,

where C – angle between sidesa And b .